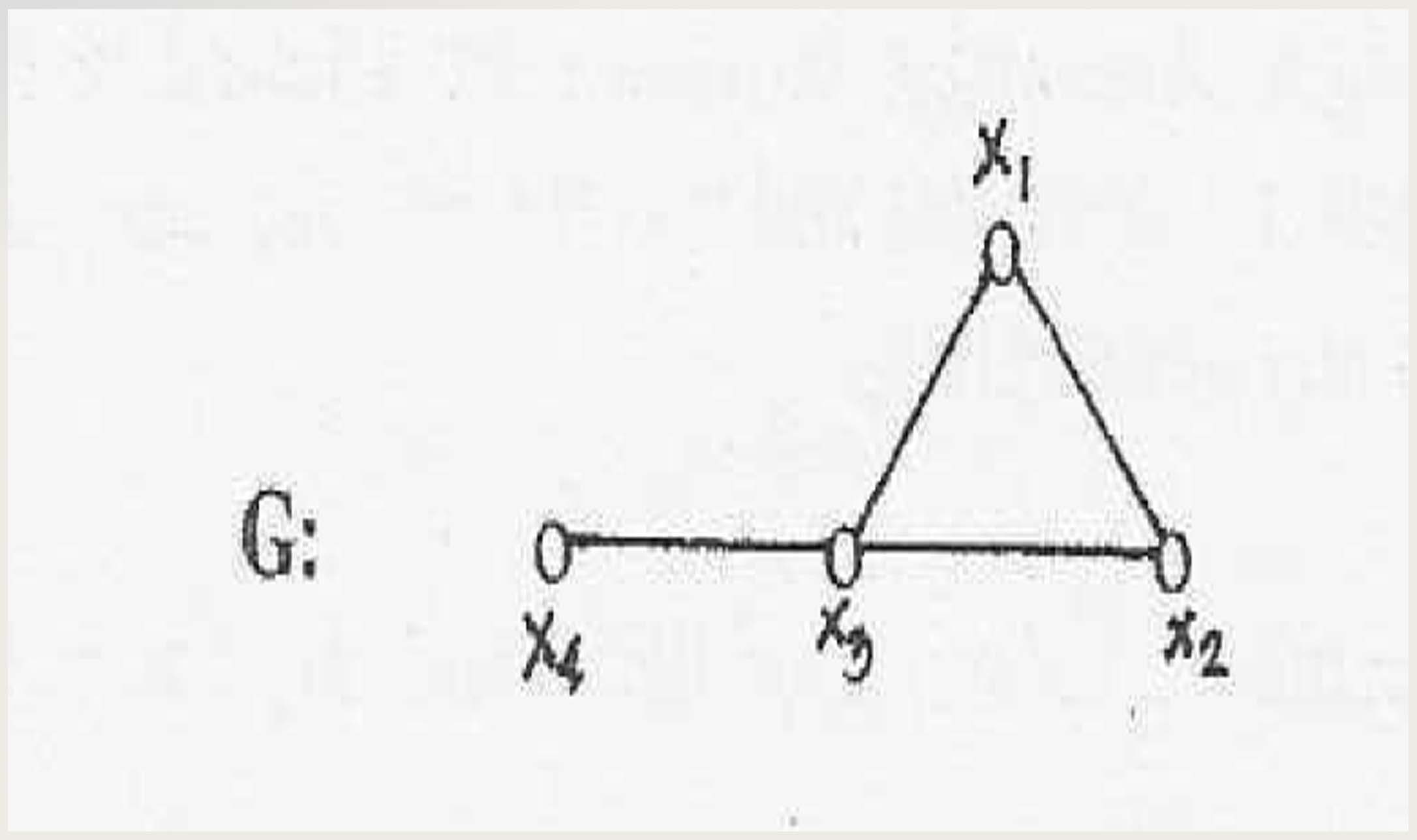
The Geometric Index of the Wheel, Wn
DOI:
https://doi.org/10.59120/drj.v1i1.83Keywords:
unit graphs, unit embeddingAbstract
The geometric index of a graph G is defined as the smallest non-negative integer n such that a graph G is a unit graph in r. Graphs considered are finite, undirected, without loops nor multiple edges. Also, edge crossings are allowed in the figures but distinct vertices must have distinct coordinates and that the line segment joining adjacent vertices must not pass through any other vertex. In this paper, the geometric index g of the wheel Wn (n 3, 4, 5,…) is discovered and proven. The results of this study may serve as a benchmark information to other researchers interested in expanding the study of geometric index on all graphs.
Downloads
References
Gervacio, S.V. (1995). Unit Graph in Euclidean n-Space. Professional Chair Lecture, De La Salle University, Manila. 5 pp.
Downloads
Published
Issue
Section
License
Copyright (c) 1998 Rowena T. Baylon

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.
DRJ is an open-access journal and the article's license is CC-BY-NC. This license allows others to distribute, remix, tweak, and build on the author's work, as long as they give credit to the original work. Authors retain the copyright and grant the journal/publisher non-exclusive publishing rights with the work simultaneously licensed under a https://creativecommons.org/licenses/by-nc/4.0/.





