Topology and Metricity of the Complex Plane
DOI:
https://doi.org/10.59120/drj.v5i1.56Keywords:
topology, metricity, complex numbers, equinumerosityAbstract
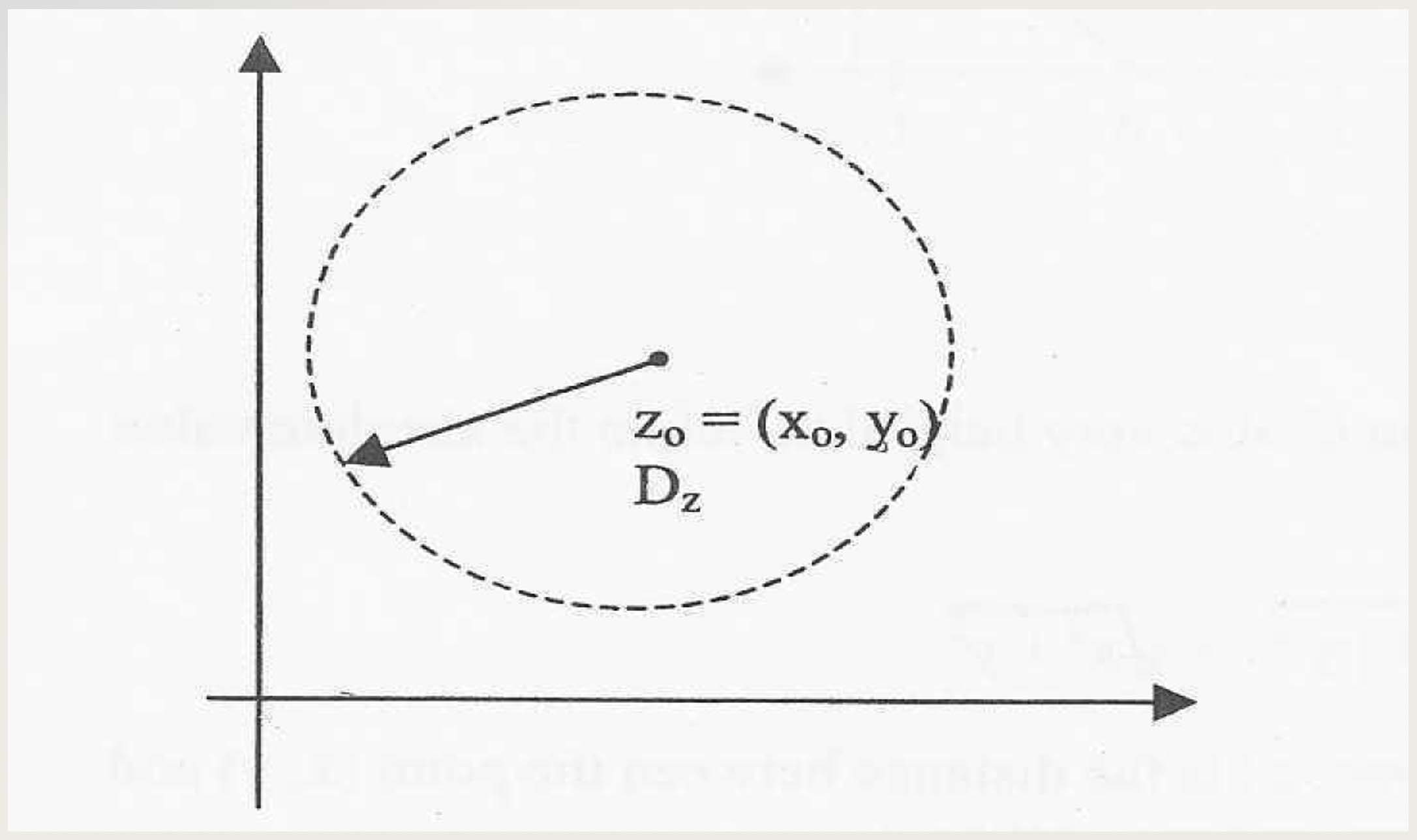
The equinumerosity of the set of complex numbers and a set of ordered pairs of real numbers is used to show the topology of the complex plane. Likewise, the metricity of the complex plane is proved with the aid of the metricity of the plane R.
Downloads
References
Churchill, R. V., & Brown, J. W. (1984). Complex Variables and Applications (4th ed.). McGraw-Hill Book Company.
Downloads
Published
Issue
Section
License
Copyright (c) 2002 Ryan C. Calungsod, Marleonie M. Bauyot

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.
DRJ is an open-access journal and the article's license is CC-BY-NC. This license allows others to distribute, remix, tweak, and build on the author's work, as long as they give credit to the original work. Authors retain the copyright and grant the journal/publisher non-exclusive publishing rights with the work simultaneously licensed under a https://creativecommons.org/licenses/by-nc/4.0/.





